Earlier today I set you five problems from Creative Puzzles to Ignite Your Mind, a book of puzzles by Shyam Sunder Gupta, former Principal Chief Engineer of Indian Railways. Here they are again with solutions.
1. Brahmagupta’s basket
The Indian mathematician Brahmagupta during the 7th century AD posed the following problem:
When eggs in a basket are taken out 2, 3, 4, 5 and 6 at a time, there remain 1, 2, 3, 4 and 5 eggs respectively. When they are taken out 7 at a time, none are leftover.
Find the smallest number of eggs that could be in the basket.
Solution 119
Let there are N eggs in the basket, so N must be divisible by 7, say N = 7m. Since on dividing N by 2, 3, 4, 5 and 6, a remainder one less than the divisor is obtained, so N + 1 must be divisible by 2, 3, 4, 5 and 6. The lowest common multiple of 2, 3, 4, 5 and 6 = 60.
N is therefore equal to some multiple of 60 minus 1, say 60k – 1. i.e. N = 60k − 1. Together with N = 7m, we can describe m in terms of k:
m = 8k + (4k – 1)/7
The value of k such that m is a positive integer is k = 2,
which gives m = 17
Hence N=119.
2. The biggest number
If the average (the mean) of 20 different positive whole numbers is 20, find the largest possible value that any one of the numbers can have.
Solution 210
Let x be the largest possible value. For x to be the largest possible number, the other 19 numbers must be as small as possible. Since all numbers are different positive integers, it is obvious that these 19 other numbers must be 1, 2, 3 … 19.
(1 + 2 + 3 +…+ 19 + x)/20 = 20
And the result follows.
3. Nine numbers
Find the missing numbers X and Y in the following list:
1100100, 10201, 1210, 400, 244, 202, X, Y, 100
Solution: X=144, Y=121
Each number in the given sequence represents the number one hundred expressed in bases 2, 3, 4, 5, 6, 7, 8, 9 and 10.
4. Match squares
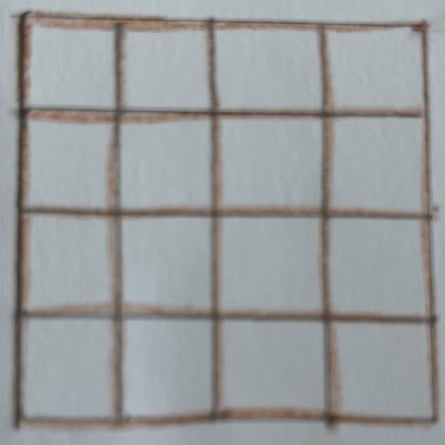
Imagine this image is of 40 match sticks arranged to make a 4×4 square.
A total of 30 squares (1 of 4×4, 4 of 3×3, 9 of 2x 2 and 16 of 1×1) can be seen in this arrangement. Find the minimum number of match sticks which on removing, vanishes all 30 squares. (i.e. the perimeter of all of them is broken.)
Solution 9 sticks
Here’s one way of doing it. The red lines are where match sticks are to be removed.
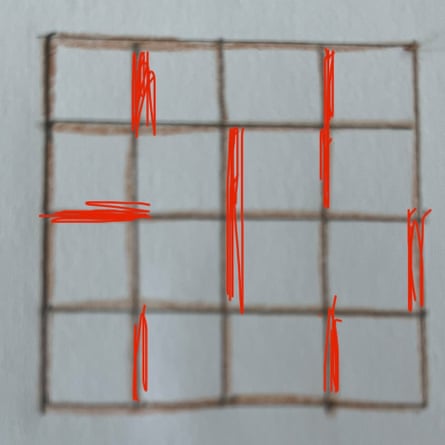
5. Square sums
Arrange the numbers from 1 to 15 in a row such that the sum of every two adjacent numbers is a square number (i.e. 1= 12, or 4= 22, or 9 = 32, etc)
Solution 9-7-2-14-11-5-4-12-13-3-6-10-15-1-8
The possible squares are 4, 9, 16 and 25, since the sum of the largest two numbers is 15 + 14 = 29, which is less than 36. We can work out the possible pairs of adjacent numbers, which sum to these squares:
4: 1+3
9: 1+8, 2+7, 3+6, 4+5
16: 1+15, 2+14, 3+13, 4+12, 5+11, 6+10, 7+9
25: 10+15, 11+14, 12+13
The number 8 is adjacent to 1 only, and number 9 is adjacent to 7 only. So, for the solution to exist, these two numbers, i.e. 9 and 8, must be placed at the ends.
Let’s start with the number 9 and put adjacent numbers 1 to 15 such that the sum of two adjacent numbers is a perfect square. 9-7-2-14-11-5-4-12-13-3
The above is the only sequence possible from 9 up to 3. From number 3 onwards, there are two possibilities: i) 3-1-8, or (ii) 3-6-10-15-1-8.
I hope you enjoyed today’s puzzles. I’ll be back in two weeks.
Thanks to Shyam Sunder Gupta. His book Creative Puzzles to Ignite Your Mind is out now. His website is www.shyamsundergupta.com.
I set a puzzle here every two weeks on a Monday. I’m always on the look-out for great puzzles. If you would like to suggest one, email me.
I give school talks about maths and puzzles (online and in person). If your school is interested please get in touch.









